mathinschool.com
numbers and algebraic expressions
logic, sets, intervals
absolute value
function and its properties
linear function
quadratic function
polynomials
rational function
exponential function
logarithm
number sequences
limits of sequences and functions
derivative and integral of functions
trigonometry
plane geometry
analytic geometry
solid geometry
combinatorics
probability
elements of statistics
kropki game
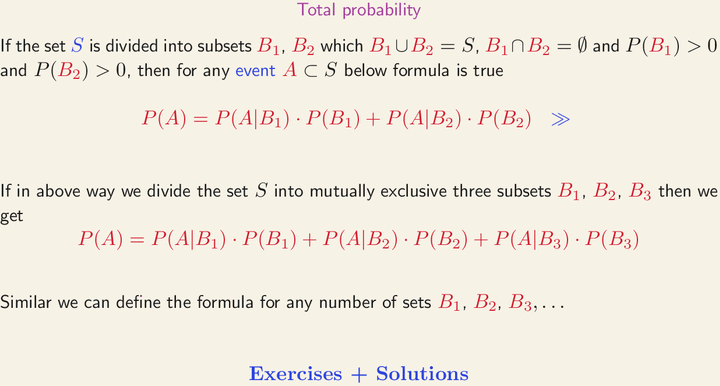
If the set S is divided into subsets B_1, B_2 which B_1 ∪ B_2 = S, B_1 ∩ B_2 = ∅ and P(B_1) gt; 0 and P(B_2) gt; 0, then for any event A ⊂ S below formula is true. P(A) = P(A|B_1) * P(B_1) + P(A|B_2) * P(B_2). If in above way we divide the set S into mutually exclusive three subsets B_1, B_2, B_3 then we get P(A) = P(A|B_1) * P(B_1) + P(A|B_2) * P(B_2) + P(A|B_3) * P(B_3). Similar we can define the formula for any number of sets B_1, B_2, B_3,...