mathinschool.com
numbers and algebraic expressions
logic, sets, intervals
absolute value
function and its properties
linear function
quadratic function
polynomials
rational function
exponential function
logarithm
number sequences
limits of sequences and functions
derivative and integral of functions
trigonometry
plane geometry
analytic geometry
solid geometry
combinatorics
probability
elements of statistics
kropki game
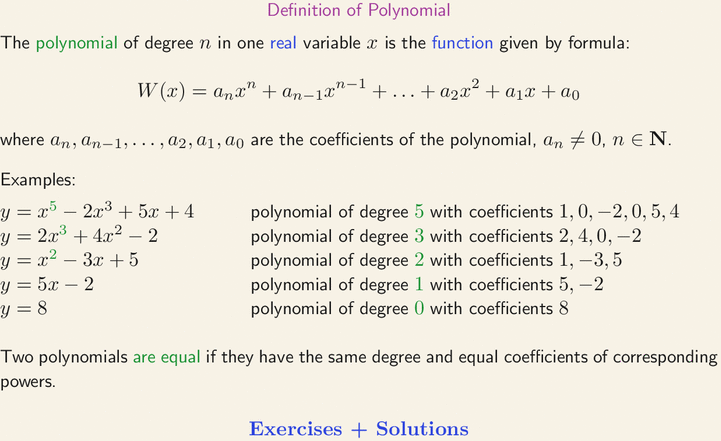
The polynomial of degree n in one real variable x is the function given by formula. W(x) = a_n x^n + a_{n-1} x^{n-1} + ... + a_2 x^2 + a_1 x + a_0 where a_n, a_{n-1}, ... , a_2, a_1, a_0 are coefficients of the polynomial, a_n ≠ 0, n ∈ N, y = x^5 - 2x^3 + 5x + 4 - polynomial of degree 5 with coefficients 1, 0, -2, 0, 5, 4. y = 2x^3 + 4x^2 - 2 - polynomial of degree 3 with coefficients 2, 4, 0, -2. y = x^2 - 3x + 5 - polynomial of degree 2 with coefficients 1, -3, 5, y = 5x-2 - polynomial of degree 1 with coefficients 5, -2. y = 8 - polynomial of degree 0 with coefficients 8. Two polynomials are equal if they have the same degree and equal coefficients of corresponding powers.